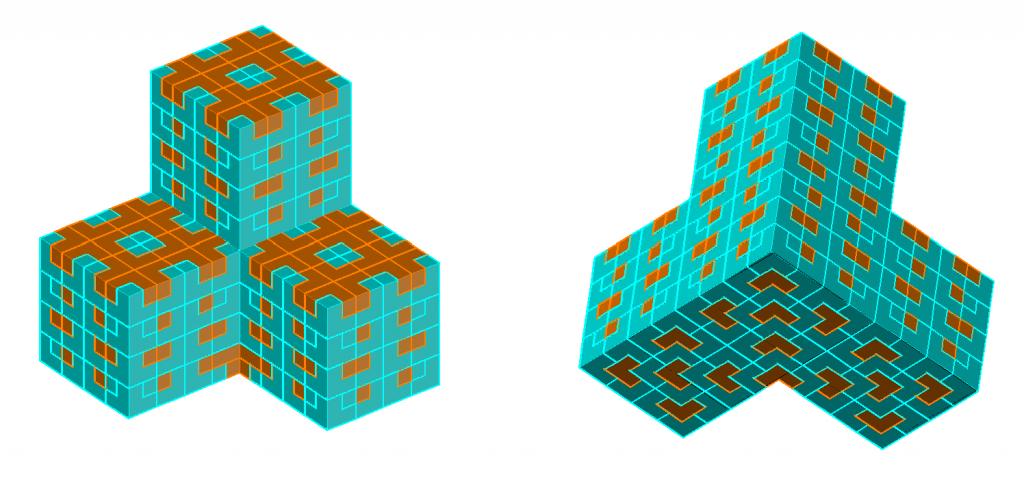
3D Chair Tiles are cubes in which a cube one eighth in size is cut out in one corner . Alternatively they may be described in terms of the smaller cubes as hepta-cube tiles. By applying a simple substitution rule one gets the well-known 3d-chair tiling.

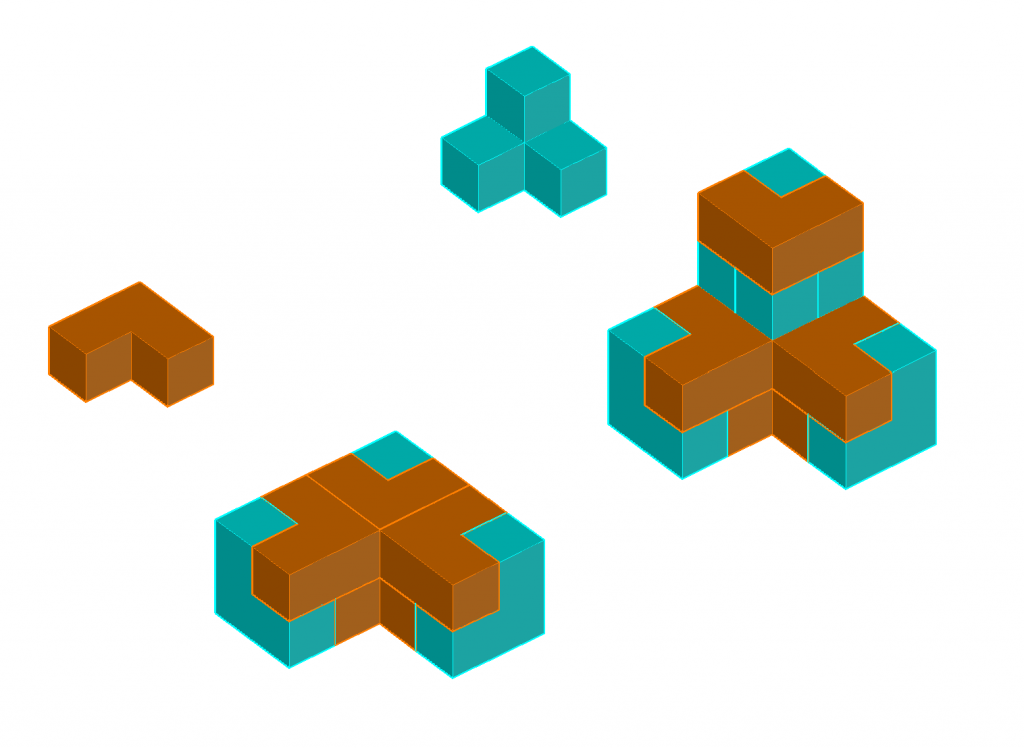
Here we will demonstrate that a similar aperiodic substitution tiling is possible based on two different polycube prototiles, i.e. the tri-cube and tetra-cube shown in figure 1.

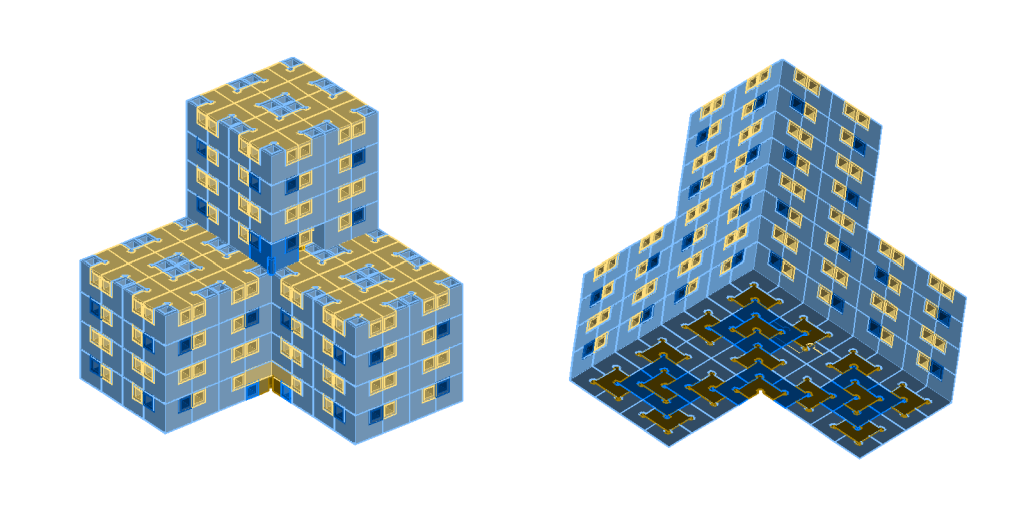
For a multiplication factor of 2, the first substitution tiles contain 24 and 32 small cubes respectively. The bottom and top face of the tricube are identical to the 2D chair tile. Therefore, a trivial tri-cube substitution tile consists of two layers of four tri-cube prototiles. Also a trivial tetra-cube supertile can be built from 8 tetra-cube prototiles by combining two of them to a basic cube. In order to exclude thes trivial solutions, we demand that the substitution tiles are built from a finite number of both types of prototiles. In fact, we choose the numbers to be four and three respectively for the super-tri-cube, and four and five for the super-tetra-cube. There are still a number of different ways to construct the supertiles. Our choise is to make them as symmetrical as possible as shown in figure 1.

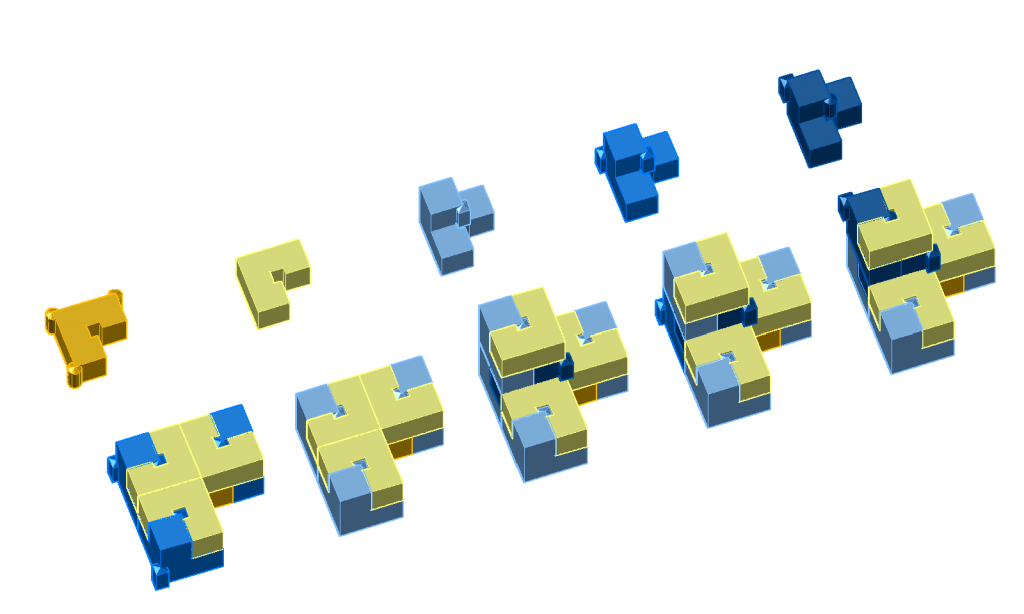
To force these supertiles to have the appearance shown in fig. 1, we distinguish two different tri-cubes and three different tetra-cubes by adding pegs and slots to some of their edges in the way shown in fig. 2.

The resulting tiling has a bottom face, which is identical to the 2D chair tiling, as is shown on the right hand side of fig. 3. Most of the super-cube faces parallel and perpendicular to the bottom face are periodic.